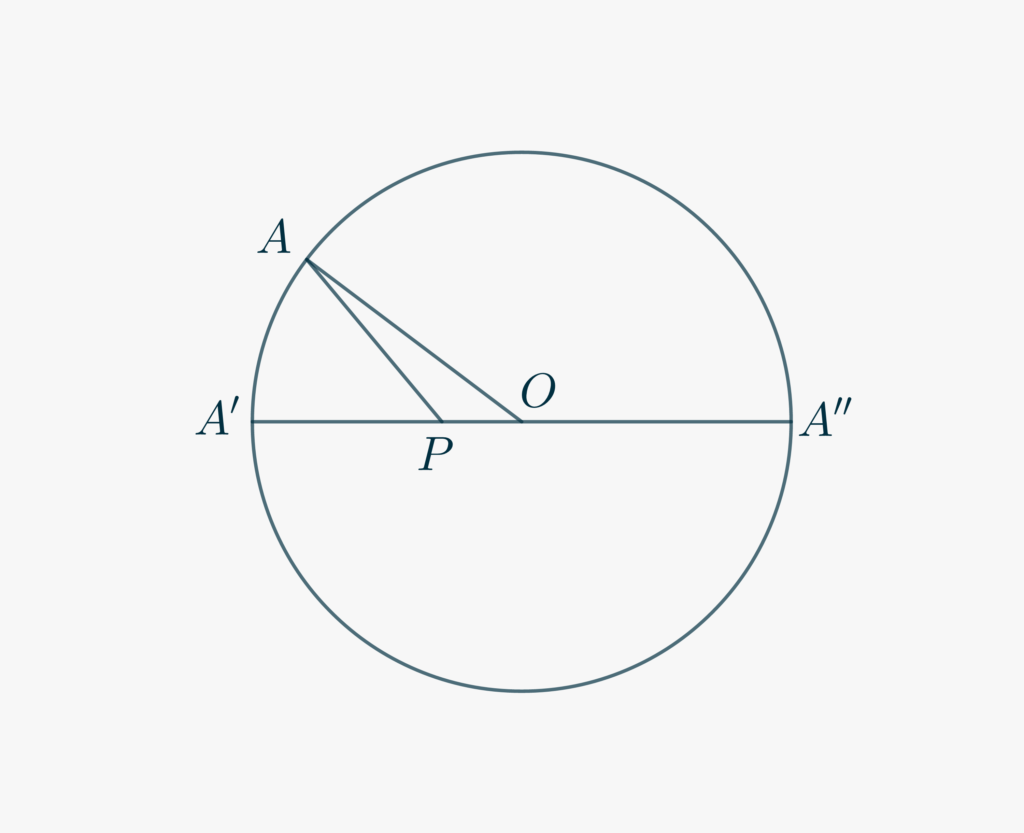
Consider a circle and a point \(P\) inside it. We know that the minimum distance between \(P\) and the points on the circumference is \(2\) cm, while the maximum distance is \(8\) cm. What is the circle radius?
As a first step, we can show that the minimum and maximum distances are reached when the points are on the intersection between the circumference and the diameter passing through \(P\).
Referring to the Figure in the bottom, note that
\begin{equation}A’P+OP = r,\tag{1}\label{eq562:uno}\end{equation}
where \(r\) is the circle radius. Furthermore, by triangular inequality on \(\triangle OAP\),
\begin{equation}AP + OP \geq r.\tag{2}\label{eq562:due}\end{equation} Comparing \eqref{eq562:uno} e \eqref{eq562:due} yields
\[AP \geq A’P.\]
Analogously,
\begin{equation}A^{\prime\prime} P=OP+r\tag{3}\label{eq562:tre}\end{equation}
while, again because of triangular inequality,
\begin{equation}AP\leq OP +r.\tag{4}\label{eq562:quattro}\end{equation}
By comparing \eqref{eq562:tre} e \eqref{eq562:quattro} we deduce that
\[AP \leq A^{\prime\prime}P.\]
Thus \(A’P = 2\) cm, \(A^{\prime\prime}P = 8\) cm, and the required radius is
\[r = \frac{A’P+A^{\prime\prime}P}{2}=5 \ \mbox{cm}\]